In the lowland rain forests of Borneo, most of the giant emergent canopy trees are dipterocarps, as for example Shorea leprosula, a tree so large that I had to crane my neck to see the crown 70 to 80 meters above my head, and I began to reflect on what the world looked like from up there, and then on all the creatures, plant and animal, that actually live in that epiphyte-draped world, and this led to thoughts that some of the creatures living up there must fall now and then, and what happens to them when they do? That was naturally and quickly followed by what it would be like for me to be up there and then make that fall. I would quickly reach a terminal velocity of about 130 miles per hour and my momentum would kill me when I came to sudden splat on the forest floor. I knew that an insect falling from that same tree would be in no danger, and having enjoyed the scenery on the way down and landed on the forest floor, it would simply go, "harumph", look around and crawl away, perhaps wondering how in the world it was ever going get back home all the way up there.
It's hard to improve on how the British biologist J. B. S. Haldane described the situation in a 1926 article in Harper’s Magazine: “To the mouse and any smaller animal [gravity] presents practically no dangers. You can drop a mouse down a thousand-yard mine shaft; and, on arriving at the bottom, it gets a slight shock and walks away. A rat is killed, a man is broken, a horse splashes. For the resistance presented to movement by the air is proportional to the surface of the moving object. Divide an animal's length, breadth, and height each by ten; its weight is reduced to a thousandth, but its surface only to a hundredth. So, the resistance to falling in the case of the small animal is relatively ten times greater than the driving force.”
This is all well and good, but so general as to be unsatisfying--- big things fall faster than little things. Really? Who knew? There are many sources that will tell you what the terminal velocity of a falling human, for example, a skydiver would be, but when it comes to little things like insects, you pretty much have to come up with the numbers yourself. Since I seem to have gotten enamored with the life of my tiny little pet ant, Pheidole adrianoi, it seemed logical and natural to ask how fast this little ant would fall, were I to drop it, not from the top of a Bornean tree, but from some, in ant relative terms, great height.
I have already written on the air resistance this ant meets when it runs, and now want to add how air resists its fall. Things that fall in a vacuum all fall at the same rate, simply adhering to the acceleration of gravity, but the fall of things in a fluid such as air is retarded by the fluid friction of the air. As Haldane wrote, friction resides in the surface of the object, whereas the “driving force”, gravity, resides in its mass. Because my little ant weighs only about 40 micrograms, I already know that its rate of fall is not going to be great.
But can I really measure this rate directly, or do I have to depend on theory and equations? I have the utmost respect for theories--- no, really, I do--- but measurements of falling ants would seem challenging. I could drop an ant in front of a video camera and see how fast it falls. With my simple equipment, my ant video attempts were non-starters. But then how about this? Drop an ant through the visual field of a camera with the lens open while illuminating the fall with a strobe light, as I did with steel balls falling through water? Every time the strobe flashes, the camera should record an image, so the interval between flashes/images should let me calculate the rate of fall. Sadly, that didn’t work either because the contrast between the ants and the background was too small.
But then, does it matter whether the object moves downward through the air or the air moves upward around the object? No, it doesn’t, so the answer to the most pressing question of 2023 “What is the terminal velocity of a Pheidole adrianoi worker falling through air at 22 degrees centigrade and one atmosphere pressure” could be gotten by suspending the ant in an upward-moving stream of air.
Among the assorted junk and cast-off lab equipment that I had accumulated over my 45 years of research were several flow meters along with assorted connectors, tubing and other detritus. Flow meters are tubes that have a slight taper from bottom to top, and a little glass or steel ball that, when you blow air in the bottom of the tube, become suspended at a position dependent on the flow rate of the air. In other words, you can read the flow rate from the position of the ball. Simple, eh?

The flow meter reads in milliliters of air per minute (cm3/min), that is, in volume, but what I really wanted to know was not the volume but the speed of the upward-moving air, and for that, I needed the cross-sectional area (cm2) of the tube all along its length. Clearly, since the tube is tapered, its cross-sectional area increases toward the top. In fact, it is this taper that determines where the ball comes to rest under a given rate of flow. So, knowing the cubic centimeters per second (cm3/sec) going up the tube, dividing that by the cross-sectional area (cm2), we arrive at the air speed in centimeters per second. Piece of cake.
The flow meter, being a pricey one, came already calibrated, and measuring the diameter at various points was easily done under magnification, so converting the scale position to air speed in cm/sec was easy.

All I needed next was a source of air. A little aquarium pump supplied the air flow for the flow meter, and a simple needle valve allowed me to control the flow rate, while my digital microscope was was set up to record the coming action.
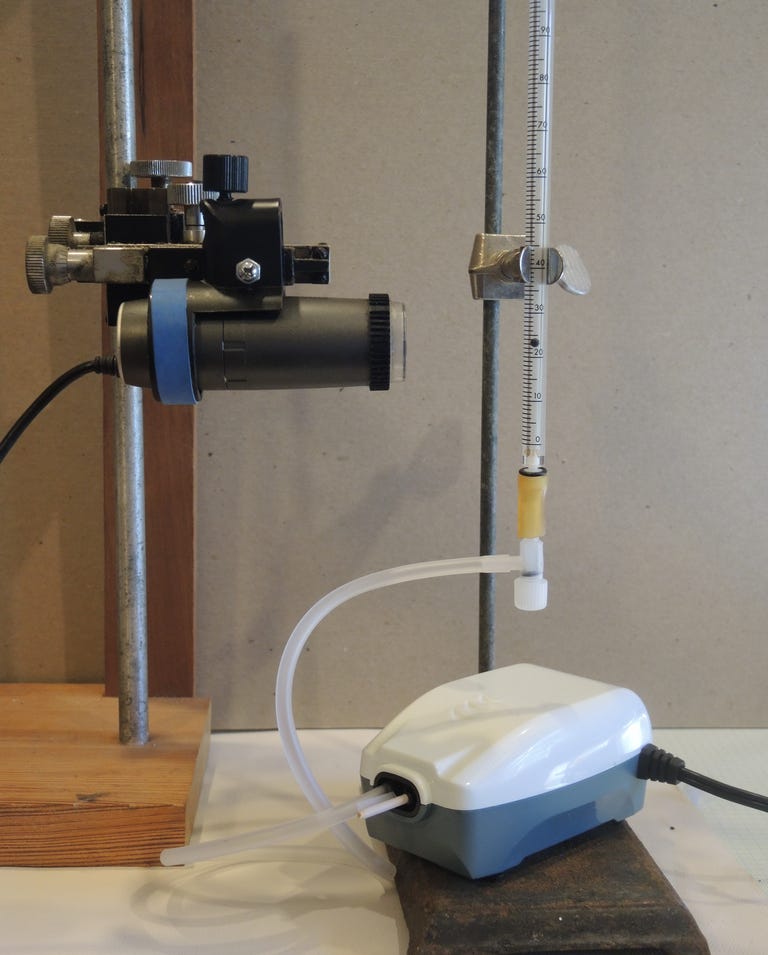
It was then a simple matter to drop a worker Pheidole adrianoi into the top of the tube and see where it comes to rest in the upward flowing air. At first I dropped in a live worker or two, but the result was comedic because the ant would tumble around until it happened to hit the wall, at which time it would grab the wall and begin to climb out. It must have been like walking in a hurricane. Dead workers lacked this ability to mess up my experiment like that, and quickly came to rest at a position in the tube.
Well, they didn’t exactly rest, as you can see in this video, but fluctuated between clear limits, bouncing between about 54 and 52 cm per second, or about 0.53 meters per second. In 10 seconds, the ant would have fallen five meters. This then is the terminal velocity the ant would reach if we dropped it from the top of that tree in Borneo. Contrast that with the terminal velocity of a human falling through air--- 55 to 90 meters per second, or more than 100 times as fast. In 10 seconds, that unfortunate man could have fallen almost a kilometer.
Why did the ant not assume a steady position in the air flow like the ball did? The fluid friction of the air depends on the “aspect” of the object, that is, the area of its projection at right angles to its direction of fall. When the ant is spread-eagled, its projected area and hence its drag is greater than, for example, when it is head-down with a much smaller projected area and drag. So as the ant flips around in the air stream, the fluid friction on its body varies a great deal and the ant bounces up and down. The ball, on the other hand, is a smooth sphere, and its projection is identical no matter how it turns and tumbles.

As gravity accelerates falling objects, they gain kinetic energy or momentum, defined as the product of their mass times their velocity. Friction with the air transfers some of that kinetic energy to the air, eventually reaching a steady state, the terminal velocity, in which the acceleration of gravity is equal to the frictional retardation of the air. This state is determined by the the ratio of the kinetic energy (momentum) to the viscous resistance of the air. The greater the size/weight of the object, the more the inertial force dominates over the fluid's frictional resistance.
Of course, an object falling through the atmosphere eventually hits the ground and stops. As the saying goes, it’s not the long fall that hurts you, it’s the sudden stop, as so effectively described by J.B.S. Haldane above. The momentum is the package of kinetic energy inherent in falling objects. To stop the fall, all that kinetic energy has to be converted to some other form of energy. When the momentum is large relative to the structural strength of the object, that conversion is a destructive, rupturing, breaking force.
Ants and people are both made of stuff with pretty similar structural strength, but our 0.04 mg ant falling at 0.5 m/sec has a momentum of only 0.00000002 kg-m/sec, whereas a 70 kg man falling through air at 50 m/sec has a momentum of 35,000 kg-m/sec, or more than 100 billion times as much as the ant. No wonder the man needs a parachute to keep him from splattering on the ground, while the ant doesn’t. In effect, the ant IS a parachute.
As you can see, the experience of falling is radically different for small and large things. Were I to fall from that Shorea leprosula tree, the fluid resistance of air would do little to slow my fall because my fall would be dominated by gravity and momentum. For a small creature like Pheidole adrianoi, its fall would be dominated by the fluid friction of air and it would never reach a velocity or momentum great enough even to cause it discomfort upon landing. Maybe that’s why we don’t live in trees.




Walter, as usual I was impressed with your essay. I was along for the ride with the falling mice and horses. However, when you got to your actual experiments and the dreaded math (for me, of course) I had the feeling of being lost in that Borneo forest. Seeking a comparison between making sense of the marvelous math and physics in your essay and my comprehension, I came up with this: I just checked out of the library The Brothers Karamazov, in English. If, however, I attempted to read in Russian (old or contemporary) I would have similar comprehension. As I have lamented to you before, I wish the hell I had paid attention in high school rather than dreaming of hot rods and girls and not in that order. Steve
Where is the assurance that “no animals were harmed the making of this experiment.” Maybe we need an essay on "how to gather dead ants for experiments", or "how to scrape dead ants off your shoes", or "efficient means of collecting experimental subjects without contaminating the remains with dog shit".”
Lots of possibilities. And, I LOVED THIS!!! just like the explanation of why the little pucks slide easily along the surface of the bowling pinball game. Backwoods of California.